Residual interaction in finite nuclei

Non-self-consistent calculations often use the residual Landau-Migdal interaction in the spin-isospin channel:

$${ v_{\rm res} (\vec{r}, \vec{r}') }$$

$$N_0 \Big[ g_0' \, \delta (\vec{r}- \vec{r}') + g_1' \, \vec{k}' \... ...sigma$}}}') \, (\mbox{{\boldmath {$\tau$}}} \cdot \mbox{{\boldmath {$\tau$}}}')$$ = (13)

where N₀ is a normalization factor [see Eq. (69)] and $\vec{k}$ and $\vec{k}'$ are defined in Appendix 8. In most applications, only the s-wave interaction with strength g₀' is used, and the matrix elements of the force are not antisymmetrized. The underlying single-particle spectra are usually taken from a parameterized potential, e.g., the Woods-Saxon potential. Typical values for g₀', obtained from fits to GT-resonance systematics, are $1.4 \leq g_0' \leq 1.6$ [45,46,47]. (See Ref. [48] for an early compilation of data.) Sometimes this approach is formulated in terms of the residual interaction between antisymmetrized states. The results are similar, e.g., g₀' = 1.54 in the double-$\beta$-decay calculations by Engel et al. [49]. More complicated residual interactions, like boson-exchange potentials, have been used as well; see, e.g., Refs. [50,51,52]. Borzov et al. use a renormalized one-pion exchange potential in connection with a $\ell = 0$ Landau-Migdal interaction of type (13) [53].

A much simpler residual interaction in the GT channel is a separable (or ``schematic") interaction, $v_{\rm res} = \kappa_{\rm GT} \; (\mbox{{\boldmath {$\sigma$}}} \cdot \mbox{{\b... ...igma$}}}' )\; (\mbox{{\boldmath {$\tau$}}} \cdot \mbox{{\boldmath {$\tau$}}}')$, where the strength $\kappa_{\rm GT}$ has to be a function of A. This interaction is widely used in global calculations of nuclear $\beta$-decay [54,55]. Sarriguren et al. [56] use it for a description of the GT resonances in deformed nuclei with quasiparticle energies obtained from self-consistent HF+BCS calculations. They estimate $\kappa_{\rm GT}$ from the Landau parameters of their Skyrme interaction. (The same prescription is used in their calculations of M1 resonances [57].) But however useful this approach may be from a technical point of view, it is not self-consistent. Nor is it equivalent to using the original residual Skyrme interaction; see, e.g., the discussion in [46].

A truly self-consistent calculation, by contrast, should interpret the QRPA as the small-amplitude limit of time-dependent HFB theory. The Skyrme energy functional used in the HFB should then determine the residual interaction between unsymmetrized states in the QRPA:

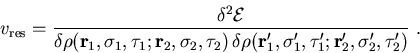$$v_{\rm res} = \frac{\delta^2 {\cal E}} {\delta \rho (\vec{r... ...r}_1', \sigma_1', \tau_1'; \vec{r}_2', \sigma_2', \tau_2')} ~.$$ (14)

The actual form of the residual interaction that contributes to the QRPA matrix elements of 1⁺ states is outlined in Appendix 12.

Table 1: Landau parameters for various Skyrme interactions from relations (34) and the Gogny forces D1 and D1s. Missing entries are zero by construction.

Force	g0	g1	g2	g0'	g1'	g2'
SkM*	0.33			0.94
SGII	0.62			0.93
SkP	-0.23	-0.18		0.06	0.97
SkI3	1.89			0.85
SkI4	1.77			0.88
SLy4	1.39			0.90
SLy5	1.14	0.24		-0.15	1.05
SLy6	1.41			0.90
SLy7	0.94	0.47		0.02	0.88
SkO	0.48			0.98
SkO'	-1.61	2.16		0.79	0.19
SkX	-0.63	0.18		0.51	0.53
D1	0.47	0.06	0.12	0.60	0.34	0.08
D1s	0.48	-0.19	0.25	0.62	0.62	-0.04