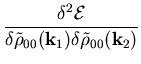Landau Parameters from the Skyrme energy functional

A simple and instructive description of the residual interaction in homogeneous INM is given by the Landau interaction developed in the context of Fermi-liquid theory [50]. Landau parameters corresponding to the Skyrme forces are discussed in Refs. [21,27,36,37,38,73]. Starting from the full density matrix in (relative) momentum space $\tilde\rho (\vec{k}\sigma \tau \sigma' \tau')$, the various densities are defined as

$$\tilde\rho_{00} (\vec{k}) \sum_{\sigma} \sum_{\tau} \tilde\rho (\vec{k}\sigma \tau \sigma \tau ) ,$$ = (55)

$$\tilde\rho_{1 t_3} (\vec{k}) \sum_{\sigma} \sum_{\tau,\tau'} \tilde\rho (\vec{k}\sigma \tau \sigma \tau' ) \; \tau^{t_3}_{\tau \tau'} ,$$ = (56)

$$\tilde{\vec{s}}_{00} (\vec{k}) \sum_{\sigma, \sigma'} \sum_{\tau} \tilde\rho (\vec{k}\sigma \tau \sigma' \tau ) \; \mbox{{\boldmath {$\sigma$}}}_{\sigma \sigma'} ,$$ = (57)

$$\tilde{\vec{s}}_{1 t_3} (\vec{k}) \sum_{\sigma, \sigma'} \sum_{\tau, \tau'} \tilde\rho (\vec{k}\sig... ... \: \mbox{{\boldmath {$\sigma$}}}_{\sigma \sigma'} \; \tau^{t_3}_{\tau \tau'} ,$$ = (58)

The kinetic densities are given by $\tau_{t t_3} = \rho_{t t_3} \, k^2$, $\vec{T}_{t t_3} = \vec{s}_{t t_3} \, k^2$. The Landau-Migdal interaction is defined as

$${ \tilde{F} (\vec{k}_1 \sigma_1 \tau_1 \sigma'_1 \tau'_1; \vec{k}_2 \sigma_2 \tau_2 \sigma'_2 \tau'_2 ) }$$

$$\frac{\delta^2 {\cal E}} {\delta \tilde\rho (\vec{k}_1 \sigma_1 \... ...ma'_1 \tau'_1) \delta \tilde\rho (\vec{k}_2 \sigma_2 \tau_2 \sigma'_2 \tau'_2)}$$ =

$$\tilde{f} (\vec{k}_1, \vec{k}_2) + \tilde{f}{}' (\vec{k}_1, \vec{... ...}}}_1 \cdot \mbox{{\boldmath {$\tau$}}}_2 \phantom{\frac{\delta^2}{\tilde\rho}}$$ =

$$+ \tilde{g} (\vec{k}_1, \vec{k}_2) \; \mbox{{\boldmath {$\sigma$}... ...ldmath {$\tau$}}}_1 \cdot \mbox{{\boldmath {$\tau$}}}_2) . \phantom{\bigg\vert}$$ (59)

The isoscalar-scalar, isovector-scalar, isoscalar-vector, and isovector-vector channels of the residual interaction are given by

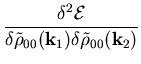$$\tilde{f} (\vec{k}_1, \vec{k}_2) \frac{\delta^2 {\cal E}} {\delta \tilde\rho_{00} (\vec{k}_1) \delta \tilde\rho_{00} (\vec{k}_2)}$$ = (60)

$$\tilde{f}{}' (\vec{k}_1, \vec{k}_2) \frac{\delta^2 {\cal E}} {\delta \tilde\rho_{1 t_3} (\vec{k}_1) \delta \tilde\rho_{1 t_3} (\vec{k}_2)}$$ = (61)

$$\tilde{g} (\vec{k}_1, \vec{k}_2) \frac{\delta^2 {\cal E}} {\delta \tilde{\vec{s}}_{00} (\vec{k}_1) \delta \tilde{\vec{s}}_{00} (\vec{k}_2)}$$ = (62)

$$\tilde{g}{}' (\vec{k}_1, \vec{k}_2) \frac{\delta^2 {\cal E}} {\delta \tilde{\vec{s}}_{1 t_3} (\vec{k}_1) \delta \tilde{\vec{s}}_{1 t_3} (\vec{k}_2)}$$ = (63)

Assuming that only states at the Fermi surface contribute, i.e., $\vert \vec{k}_1 \vert = \vert \vec{k}_2 \vert = k_{\rm F}$, $\tilde{f}$, $\tilde{f}{}'$, $\tilde{g}$, and $\tilde{g}{}'$ depend on the angle $\theta$ between $\vec{k}$ and $\vec{k}$ only, and can be expanded into Legendre polynomials, e.g.

$$\tilde{f} (\vec{k}_1, \vec{k}_2) = \frac{1}{N_0} \sum_{\ell = 0}^{\infty} f_\ell \; P_\ell (\theta) .$$ (64)

The normalization factor N₀ is the level density at the Fermi surface

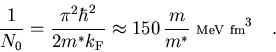$$\frac{1}{N_0} = \frac{\pi^2 \hbar^2}{2 m^* k_{\rm F} } \appr... ...\rm\scriptsize {MeV}} \; \mbox{\rm\scriptsize {fm}}^3 \quad .$$ (65)

A variety of definitions of the normalization factor N₀ are used in the literature and great care has to be taken when comparing values from different groups; see, e.g., Ref.[50] for a detailed discussion. We use the convention defined in [38]. The Landau parameters corresponding to the general energy functional (6) are

$$N_0 \left( 2 C_0^{\rho} + 4 \frac{\partial C_0^{\rho}}{\partial \... ...tial \rho_{00}^2} \rho_0^2 + 2 C_0^{\tau} \, \beta \, \rho_{00}^{2/3} \right) ,$$ f₀ =

$$N_0 \big( 2 C_1^{\rho} + 2 C_1^{\tau} \, \beta \, \rho_{00}^{2/3} \big) ,$$ f₀' =

$$N_0 \big( 2 C_0^{s} + 2 C_0^{T} \, \beta \, \rho_{00}^{2/3} \big) ,$$ g₀ =

$$N_0 \big( 2 C_1^{s} + 2 C_1^{T} \, \beta \, \rho_{00}^{2/3} \big) ,$$ g₀ =

$$- 2 N_0 \; C_0^{\tau} \, \beta \, \rho_{00}^{2/3} ,$$ f₁ =

$$- 2 N_0 \; C_1^{\tau} \, \beta \, \rho_0^{2/3} ,$$ f₁' =

$$- 2 N_0 \; C_0^{T} \, \beta \, \rho_{00}^{2/3},$$ g₁ =

$$- 2 N_0 \; C_1^{T} \, \beta \, \rho_{00}^{2/3}.$$ g₁' = (66)

Higher-order Landau parameters vanish for the second-order energy functional (12), but not for finite-range interactions as the Gogny force discussed in the next Appendix. The Landau parameters provide a stability criterion for symmetric unpolarized INM: It becomes unstable for a given interaction when either $f_\ell$, $f_\ell'$, $g_\ell$, or $g_\ell'$ is less than $-(2 \ell + 1)$.