Energy density functional from the two-body Skyrme force

Table 4: Time-odd coupling constants calculated from Eq. (34) for the Skyrme interactions as indicated.

Force	C0s[0]	C1s[0]	$C_0^{s}[\rho_{\rm nm}]$	$C_1^{s} [\rho _{\rm nm}]$	C0T	C1T	$C_0^{\Delta s}$	$C_1^{\Delta s}$	$\alpha$
	$({\rm MeV}\, {\rm fm}^3)$	$({\rm MeV}\, {\rm fm}^3)$	$({\rm MeV}\, {\rm fm}^3)$	$({\rm MeV}\, {\rm fm}^3)$	$({\rm MeV}\, {\rm fm}^5)$	$({\rm MeV}\, {\rm fm}^5)$	$({\rm MeV}\, {\rm fm}^5)$	$({\rm MeV}\, {\rm fm}^5)$
SkI1	695.860	239.200	120.190	99.573	0.0	0.0	192.660	62.766	1/4
SkI3	84.486	220.360	253.180	113.940	0.0	0.0	92.235	22.777	1/4
SkI4	44.038	231.980	209.030	104.120	0.0	0.0	124.590	37.943	1/4
SkO	373.770	262.960	41.421	84.253	0.0	0.0	70.365	26.590	1/4
SkO'	277.910	262.430	47.082	84.154	-104.090	-9.172	42.791	16.553	1/4
SkX	57.812	180.660	-35.639	81.246	-7.861	-23.669	-4.434	9.514	1/2
SGII	271.110	330.620	61.048	91.676	0.0	0.0	15.291	15.283	1/6
SkP	152.340	366.460	-31.328	78.562	7.713	-41.127	-4.211	9.757	1/6
SkM*	271.110	330.620	31.674	91.187	0.0	0.0	17.109	17.109	1/6
SLy4	-207.820	311.110	153.210	99.737	0.0	0.0	47.048	14.282	1/6
SLy5	-171.360	310.430	151.080	99.133	-14.659	-65.058	45.787	14.000	1/6
SLy6	-201.460	309.940	157.050	100.280	0.0	0.0	48.822	14.655	1/6
SLy7	-215.830	310.100	158.260	100.640	-30.079	-55.951	49.680	14.843	1/6

The standard two-body Skyrme force is given by [2,33]

$${v_{\rm Skyrme} (\vec{r}_1, \vec{r}_2)}$$

$$t_0 \, ( 1 + x_0 \hat{P}_\sigma ) \; \delta (\vec{r}_1 - \vec{r}_2)$$ =

$$+ {\textstyle\frac{{1}}{{2}}} \; t_1 \; ( 1 + x_1 \hat{P}_\sigma ... ...r}'_1 - \vec{r}'_2) + \delta (\vec{r}_1 - \vec{r}_2) \; \hat{\vec{k}}{}^2 \Big]$$

$$+ t_2 \, ( 1 + x_2 \hat{P}_\sigma ) \; \hat{\vec{k}}{}' \cdot \delta (\vec{r}_1 - \vec{r}_2) \; \hat{\vec{k}}$$

$$+ {\textstyle\frac{{1}}{{6}}} \, t_3 \; ( 1 + x_3 \hat{P}_\sigma ... ...) \; \rho^\alpha \left( {\textstyle\frac{{\vec{r}_1 + \vec{r}_2}}{{2}}} \right)$$

$$+ \mbox{\rm\scriptsize {i}}W_0 \, ( \hat{\mbox{{\boldmath {$\sigm... ...\hat{\vec{k}}{}' \times \delta (\vec{r}_1 - \vec{r}_2) \; \hat{\vec{k}} \quad ,$$ (32)

where $\hat{P}_\sigma = {\textstyle\frac{{1}}{{2}}}(1 + \hat{\mbox{{\boldmath {$\sigma$}}}}_1 \cdot \hat{\mbox{{\boldmath {$\sigma$}}}}_2$) is the spin-exchange operator, $\hat{\vec{k}} = - {\textstyle\frac{{\mbox{\rm\scriptsize {i}}}}{{2}}} (\nabla_1 - \nabla_2)$ acts to the right, and $\hat{\vec{k}}{}' = {\textstyle\frac{{\mbox{\rm\scriptsize {i}}}}{{2}}} (\nabla_1' - \nabla_2')$ acts to the left. Calculating the Hartree-Fock expectation value from this force yields the energy functional given in Eq. (12) with the coupling constants:

$$C_0^\rho {\textstyle\frac{{3}}{{8}}} t_0 + {\textstyle\frac{{3}}{{48}}} t_3 \, \rho_0^\alpha$$ =

$$C_1^\rho - {\textstyle\frac{{1}}{{4}}} t_0 \Big( {\textstyle\frac{{1}}{{2}... ...{{1}}{{24}}} t_3 \Big( {\textstyle\frac{{1}}{{2}}} + x_3 \Big) \, \rho_0^\alpha$$ =

$$- {\textstyle\frac{{1}}{{4}}} t_0 \Big( {\textstyle\frac{{1}}{{2}... ...{{1}}{{24}}} t_3 \Big( {\textstyle\frac{{1}}{{2}}} - x_3 \Big) \, \rho_0^\alpha$$ C₀^s =

$$- {\textstyle\frac{{1}}{{8}}} t_0 - {\textstyle\frac{{1}}{{48}}} t_3 \, \rho_0^\alpha$$ C₁^s =

$$C_0^\tau {\textstyle\frac{{3}}{{16}}} \, t_1 + {\textstyle\frac{{1}}{{4}}} t_2 \; \Big( {\textstyle\frac{{5}}{{4}}} + x_2 \Big)$$ =

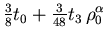$$C_1^\tau - {\textstyle\frac{{1}}{{8}}} t_1 \Big({\textstyle\frac{{1}}{{2}}... ... + {\textstyle\frac{{1}}{{8}}} t_2 \Big({\textstyle\frac{{1}}{{2}}} + x_2 \Big)$$ =

$$\eta_J \, \Big[ - {\textstyle\frac{{1}}{{8}}} t_1 \Big( {\textsty... ...xtstyle\frac{{1}}{{8}}} t_2 \Big( {\textstyle\frac{{1}}{{2}}} + x_2 \Big) \Big]$$ C₀^T =

$$\eta_J \, \Big[ - {\textstyle\frac{{1}}{{16}}} t_1 + {\textstyle\frac{{1}}{{16}}} t_2 \Big]$$ C₁^T =

$$C_0^{\Delta \rho} - {\textstyle\frac{{9}}{{64}}} t_1 + {\textstyle\frac{{1}}{{16}}} t_2 \Big( {\textstyle\frac{{5}}{{4}}} + x_2 \Big)$$ =

$$C_1^{\Delta \rho} {\textstyle\frac{{3}}{{32}}} t_1 \Big( {\textstyle\frac{{1}}{{2}}... ... {\textstyle\frac{{1}}{{32}}} t_2 \Big( {\textstyle\frac{{1}}{{2}}} + x_2 \Big)$$ =

$$C_0^{\Delta s} {\textstyle\frac{{3}}{{32}}} t_1 \Big( {\textstyle\frac{{1}}{{2}}... ... {\textstyle\frac{{1}}{{32}}} t_2 \Big( {\textstyle\frac{{1}}{{2}}} + x_2 \Big)$$ =

$$C_1^{\Delta s} {\textstyle\frac{{3}}{{64}}} t_1 + {\textstyle\frac{{1}}{{64}}} t_2$$ =

$$C_0^{\nabla J} - {\textstyle\frac{{3}}{{4}}} W_0$$ =

$$C_1^{\nabla J} - {\textstyle\frac{{1}}{{4}}} W_0$$ =

$$C_0^{\nabla s}$$ = 0

$$C_1^{\nabla s}$$ = 0 , (33)

nine of which are independent. Although in this approach $\eta_J = 1$, many parameterizations of the Skyrme interaction set $\eta_J = 0$. That violates the interpretation of the Skyrme functional as an expectation value of a real two-body interaction and removes the rationale for calculating the time-odd coupling constants from (34). For Skyrme interactions with a generalized spin-orbit interaction [42], e.g. for SkI3, SkI4, SkO, or SkO', the spin-orbit coupling constants are given by

$$C_0^{\nabla J} = - b_4 - {\textstyle\frac{{1}}{{2}}}b_4' \qq... ...uad C_1^{\nabla J} = - {\textstyle\frac{{1}}{{2}}}b_4' \quad .$$ (34)

The resulting terms in the energy functional again cannot be represented as the HF expectation value of a two-body spin-orbit potential (see, e.g., [41]), again violating the assumptions behind the calculation of the time-odd coupling constants in (34).

As Eqs. (34) represent the standard approach to the time-odd coupling constants, it is worthwhile to take a look at the actual values. Table 4 compares them for several Skyrme forces. None of these parameterizations was obtained from observables sensitive to the time-odd terms in the energy functional. Differences among the forces merely reflect various strategies for adjusting the time-even coupling constants. Values of the density-dependent isoscalar coupling constants C₀^s, either at $\rho_0 = 0$ or at $\rho_0 = \rho_{\rm nm}$, are scattered in a wide range. This is probably one of the main sources of differences in the predictions of the forces for time-odd corrections to rotational bands. For the SLyx forces, C₀^s [0] is negative, which is unusual; most often this part of the isoscalar spin-spin interaction is repulsive at all densities. The difference will probably cause visible differences in rotational properties whenever the spin density is large at the surface. All the forces agree on the isovector coupling constant C₁^s, especially at the saturation density, i.e., $C_1^s[\rho_{\rm nm}] \approx 100 \;$MeV fm³. This simply follows from the fact that, assuming Eq. (34), C₁^s is proportional to the time-even $C_0^{\rho}$ that is fixed from binding energies and radii.