Study of $C_1^{\Delta s}$.

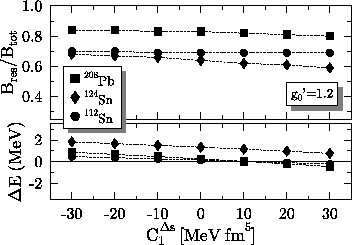
Figure 9: Variation of the GT resonance energy and the strength in the resonance when $C_1^{\Delta s}$ is varied. Symbols and scales are as in Fig. 6.

The term $C_1^{\Delta s} \vec{s}_{1t_3} \cdot \Delta \vec{s}_{1t_3}$ is sensitive to spatial variations of the isovector spin density. Unlike its (isoscalar) time-even counterpart $C_0^{\Delta \rho} \rho_0 \Delta \rho_0$, it should not be called a ``surface term'' because the spatial distribution of $\vec{s}$ is determined by a few single-particle states that do not necessarily vary the most at the nuclear surface. In discussing the effects of this term, we continue to fix C₁^T at its Skyrme-force value via gauge invariance and choose C₁^s to be density-independent and fixed from Eq. (19) with $g_0' [\rho_{\rm nm}] = 1.2$. We then vary $C_1^{\Delta s}$ over the range of $\pm 30 \, {\rm MeV}\, {\rm fm}^5$, covering the values obtained from the original Skyrme forces. As seen in Fig. 9, an increase of $C_1^{\Delta s}$ by $30 \, {\rm MeV}\, {\rm fm}^5$ has nearly the same effect on the GT resonance energies as a decrease of g₀' by 0.2, again demonstrating that the value of g₀' does not completely characterize the residual interaction in finite nuclei. A new feature of $C_1^{\Delta s}$, apparent from the curves for ¹¹²Sn and ²⁰⁸Pb in Fig. 9, is the ability to move the resonance around in energy without changing its strength.