Discussion

Mathematics behind the MP algorithm looks complicated, but to understand and use the results of the resulting parametrization equation (1) is practically sufficient. In MP with Gabor dictionary, each of the functions gi from expansion (1) is characterized by the frequency and phase (of modulation), time center and width (of the Gaussian envelope), and amplitude (related to 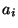$a_i$ from (1)). Expansion (1) can be interpreted as a parametric description of the signal's structures--at least those coherent with the dictionary. Presented examples of MP-based analysis of sleep EEG, intracranial epileptic recordings and event-related potentials illustrate some of the advantages offered by MP parametrization of EEG³:

• Description of signal's structures in terms of time-frequency parameters provides a direct link to the language used for standardization of visual analysis.
• Algorithm's adaptivity to local signal's structures makes possible description of relatively weak transients (usually lost in global optimizations) and gives a high time-frequency resolution.
• MP description is also fully compatible with the Fourier transform--the second widely used method of EEG analysis (Fourier basis is a part of each dictionary used for MP decomposition).
• Due to a priori elimination of cross-terms, MP estimate of time-frequency energy distribution is robust, i.e. does not produce strong artifacts at coordinates where no activity occurs in the signal.
• MP parametrization can be used, without signal-dependent tuning of procedure's parameters⁴, in a variety of problems, traditionally addressed by a variety of methods ranging from visual scoring of transients to time-frequency distributions and spectral estimates.

For a balanced picture, we should also discuss some possible drawbacks and pitfalls:

• Greedy MP algorithm requires relatively large computational resources, especially when used with stochastic dictionaries, where a priori lack of structure prevents most of the numerical optimizations [3]. However, in some cases computational complexity can be significantly reduced by a proper choice of resolutions, i.e. sizes of the dictionary, for a given problem. Rapid progress in computer technology significantly reduces the importance of computational efficiency. Finally, advances in mathematics may lead to bias-free and computationally effective algorithms.
• At present, time-frequency dictionaries are limited to structures of constant frequency and Gaussian amplitude modulation. Extensions, incorporating e.g. exponential damping or chirps, were already proposed (e.g. [7]). However, their application in EEG analysis does not seem to promise a qualitative improvement, although we'll never know until somebody really tries.
• Each derivation is analyzed separately. We'll probably have to wait at least few years for a reasonable multichannel MP, applicable in EEG research. Currently the spatial information can be retrieved by comparison of decompositions for different derivations (like e.g. in Figure 6 or [5]).

Most of the above mentioned drawbacks seem to be of transitory nature. In spite of them, MP already seems to be the best candidate for a universal method of parametrization of EEG. Description of the signal, offered by this algorithm, is suitable for analysis of both rhythmic (stationary) and transient brain activities, encompassing properties of spectral and visual EEG analysis.

Footnotes

... EEG³

More detailed discussion of some of these features can be found e.g. in [3] and [5]

... parameters⁴

Results given by most of the time-frequency methods depend heavily on the chosen settings, like e.g. choice of the mother wavelet, the length of time window, or various smoothing parameters governing the tradeoff between resolution and cross-terms contamination in quadratic distributions.