


Next: Quantum Chromodynamics (QCD)
Up: QUANTUM FIELDS OF NUCLEAR
Previous: Minute No. 4, the
Quantum Electrodynamics (QED)
Classical Jac75 and quantum Itz80 electrodynamics
are probably the best established theories of our world. They
describe interactions between charged objects, where by the charge we
mean the traditional electric charge. Quantum electrodynamics (QED)
allows to calculate electrodynamic properties of particles to an
unbelievable precision, e.g., the magnetic moment of the electron,
calculated up to the eighth order of the perturbation theory
Hug99, and the measured value Dyc87,
are in excellent agreement. Moreover, the error of the theoretical
value comes mostly from the uncertainty in the measured value of the fine
structure constant  (the first error), and less from estimated
higher-order effects (the second error).
(the first error), and less from estimated
higher-order effects (the second error).
For an electron coupled to the electromagnetic field, the Lagrangian
density, from which everything can be derived, reads
![\begin{displaymath}
{\cal L} = -{\textstyle{\frac{1}{4}}}F_{\mu\nu}F^{\mu\nu}
-...
...ma^\mu[\partial_\mu+ieA_\mu]\psi_e
- m_e \bar{\psi}_e\psi_e .
\end{displaymath}](img56.png) |
(16) |
It is expressed within the relativistic formalism that uses
space-time four-coordinates numbered by indices  =0,1,2,3.
Moreover, we assume that each pair of repeated indices implies
summation over them. Here and bellow we use the units defined by
=0,1,2,3.
Moreover, we assume that each pair of repeated indices implies
summation over them. Here and bellow we use the units defined by
 , for which the elementary charge,
, for which the elementary charge,
 ,
is a dimensionless quantity depending on the fine-structure constant
,
is a dimensionless quantity depending on the fine-structure constant
 . (Note that the elementary charge
. (Note that the elementary charge  is
positive, while the charge of the electron
is
positive, while the charge of the electron  =
= is negative.) In
such a unit system, the only unit left is the energy, so for example,
the momentum has the unit of energy, position and time - the unit of
(energy)
is negative.) In
such a unit system, the only unit left is the energy, so for example,
the momentum has the unit of energy, position and time - the unit of
(energy) , and the Lagrangian density
, and the Lagrangian density
![$[{\cal{L}}]$](img65.png) =(energy)
=(energy) (when
(when  is integrated over the
space-time it gives the dimensionless action
is integrated over the
space-time it gives the dimensionless action  ).
).
The first term in the QED Lagrangian density (16) describes
the free electromagnetic field defined by the four-potential
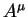

 , containing the standard scalar
(Coulomb) potential
, containing the standard scalar
(Coulomb) potential  and vector potential
and vector potential
 .
The electromagnetic field tensor
.
The electromagnetic field tensor  is defined as
is defined as
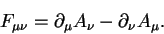 |
(17) |
The Euler-Lagrange equations corresponding to this term
give the Maxwell equations in free space, i.e., all properties
of electromagnetic waves.
The last term in (16) describes the free electron of mass
 at rest. Its field
at rest. Its field  has the structure of the
four-component Dirac spinor, but traditionally we do not explicitly
show in Lagrangian densities the corresponding indices. The first
member of the middle term (the one with
has the structure of the
four-component Dirac spinor, but traditionally we do not explicitly
show in Lagrangian densities the corresponding indices. The first
member of the middle term (the one with  ) describes the
kinetic energy of the electron, and together with the mass term, they
constitute the Lagrangian density of a free electron. The
corresponding Euler-Lagrange equations give the Dirac equation, i.e.,
all plane-wave propagation of an electron (and positron) in an
otherwise empty space. The kinetic term contains the
Dirac 4
) describes the
kinetic energy of the electron, and together with the mass term, they
constitute the Lagrangian density of a free electron. The
corresponding Euler-Lagrange equations give the Dirac equation, i.e.,
all plane-wave propagation of an electron (and positron) in an
otherwise empty space. The kinetic term contains the
Dirac 4 4 matrices
4 matrices  defined by
defined by
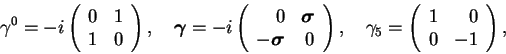 |
(18) |
where the standard Pauli 2 2 matrices
2 matrices
 read
read
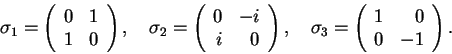 |
(19) |
Finally, the second member of the middle term in (16)
describes interaction of the electron with the electromagnetic field.
On the one hand, when considered together with the free-electron
Lagrangian it gives the Lorentz force that acts on the
electromagnetic four-current of the electron,
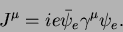 |
(20) |
On the other hand, when
considered together with the free-electromagnetic-field Lagrangian, it
gives the source terms in the Maxwell equations that correspond to
the same electron current  . The structure of the middle term
is dictated by the local gauge invariance of the QED Lagrangian
density, i.e., invariance with respect to multiplying the electron
field by a position-dependent phase. Such a local gauge invariance is
at the heart of constructing the Lagrangian densities for all
quantum-field theories applicable to the real world. We shall not
discuss these aspects during the present course.
. The structure of the middle term
is dictated by the local gauge invariance of the QED Lagrangian
density, i.e., invariance with respect to multiplying the electron
field by a position-dependent phase. Such a local gauge invariance is
at the heart of constructing the Lagrangian densities for all
quantum-field theories applicable to the real world. We shall not
discuss these aspects during the present course.
Although we only verbally described the role of each term in the QED
Lagrangian density (16), derivation and application of the
Euler-Lagrange equations is a standard route. However long, painful,
and complicated this route might be, it is a well-paved and marked
way to get physical answers. In practice, it has already been
followed way up, towards incredibly remote summits.



Next: Quantum Chromodynamics (QCD)
Up: QUANTUM FIELDS OF NUCLEAR
Previous: Minute No. 4, the
Jacek Dobaczewski
2003-01-27
![]()
![]()
![]() , containing the standard scalar
(Coulomb) potential
, containing the standard scalar
(Coulomb) potential ![]() and vector potential
and vector potential
![]() .
The electromagnetic field tensor
.
The electromagnetic field tensor ![]() is defined as
is defined as
![]() at rest. Its field
at rest. Its field ![]() has the structure of the
four-component Dirac spinor, but traditionally we do not explicitly
show in Lagrangian densities the corresponding indices. The first
member of the middle term (the one with
has the structure of the
four-component Dirac spinor, but traditionally we do not explicitly
show in Lagrangian densities the corresponding indices. The first
member of the middle term (the one with ![]() ) describes the
kinetic energy of the electron, and together with the mass term, they
constitute the Lagrangian density of a free electron. The
corresponding Euler-Lagrange equations give the Dirac equation, i.e.,
all plane-wave propagation of an electron (and positron) in an
otherwise empty space. The kinetic term contains the
Dirac 4
) describes the
kinetic energy of the electron, and together with the mass term, they
constitute the Lagrangian density of a free electron. The
corresponding Euler-Lagrange equations give the Dirac equation, i.e.,
all plane-wave propagation of an electron (and positron) in an
otherwise empty space. The kinetic term contains the
Dirac 4![]() 4 matrices
4 matrices ![]() defined by
defined by