Figures

  

Figure 1: Neutron single-particle routhians in the magic SD configuration of ³²S calculated within the HF theory for the Skyrme SLy4 interaction. Lines denoting the four (parity, signature) combinations are: solid (+,+i), dot-dashed (+,-i), dotted (-,+i), and dashed (-,-i). Standard Nilsson labels are determined by finding the dominating HO components of the HF wave-functions at low (left set) and high (right set) rotational frequencies.

  

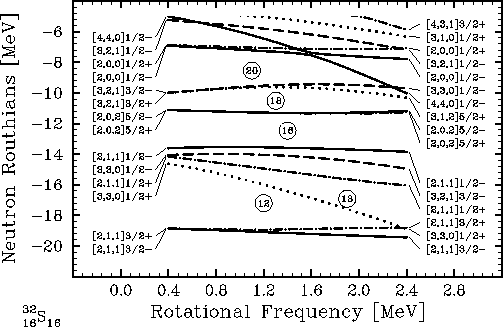Figure 2: Same as Fig. 1, but for the proton single-particle routhians.

  

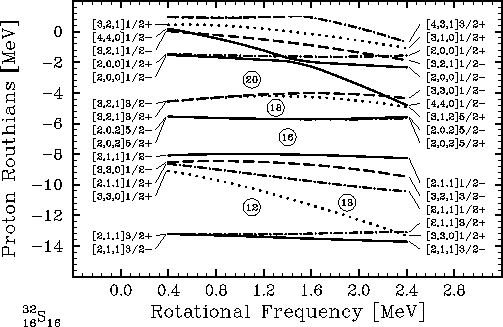Figure: Schematic diagram illustrating the single-particle neutron or proton orbitals (top), and the corresponding many-particle configurations (bottom), relevant for the description of SD bands in ³²S. The top part gives the Nilsson labels and signatures (r=$\pm {i}$), inside the circles, of orbitals on both sides of the N=16 or Z=16 Fermi level. Four labels on the left-hand side represent the N₀=3 intruder states (negative parity), and four on the right-hand side represent positive-parity states. In the bottom part, the full circles stand for occupied, the open circles for empty states. Symbols 3^n/p give numbers n or p of (neutron or proton) occupied intruder states. Subscripts $\pm$ indicate whether the number of particles in the positive-parity r=+iorbitals is larger than that in r=-i orbitals, or vice versa.

  

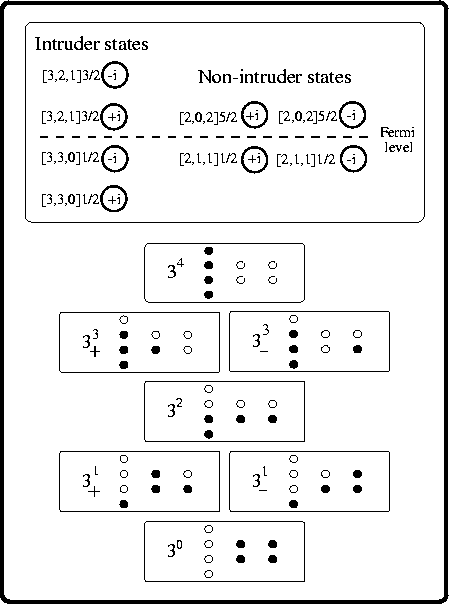Figure 4: Same as Fig. 1, but for the HF solution with the 3¹_-3¹_- configuration.

  

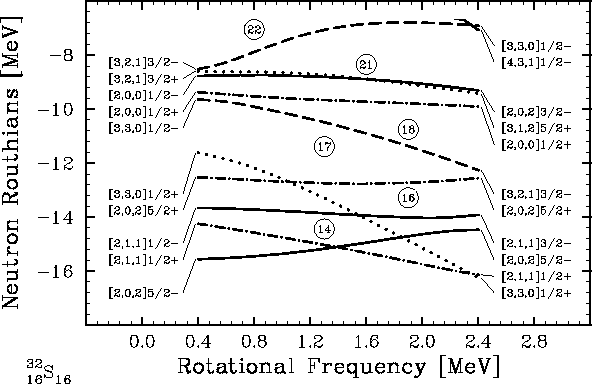Figure 5: Same as Fig. 1, but for the HF solution with the 3¹₊3¹₊ configuration.

  

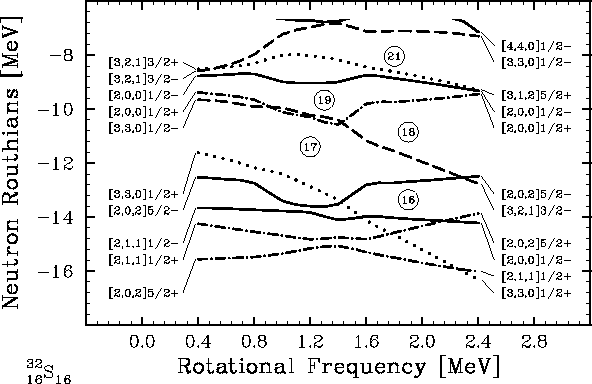Figure: Energies of the HF bands in ³²S as functions of the angular momentum. A rigid-rotor reference energy of 0.05I(I+1)MeV has been subtracted to increase clarity of the plot. Full and open symbols represent the positive- ($\pi$=+1) and negative-parity ($\pi$=-1) bands. Long-short-dashed, solid, dotted, and dashed lines correspond to neutron (r_n) and proton (r_p) signatures being equal to, respectively, (r_n,r_p)=(+,+), (+,-), (-,+), and (-,-). Note that for even numbers of protons and neutrons the possible total signatures are $r_n=\pm 1$ and $r_p=\pm 1$; the latter should not be confused with the single-nucleon signatures taking the possible values of $\pm {i}$.

  

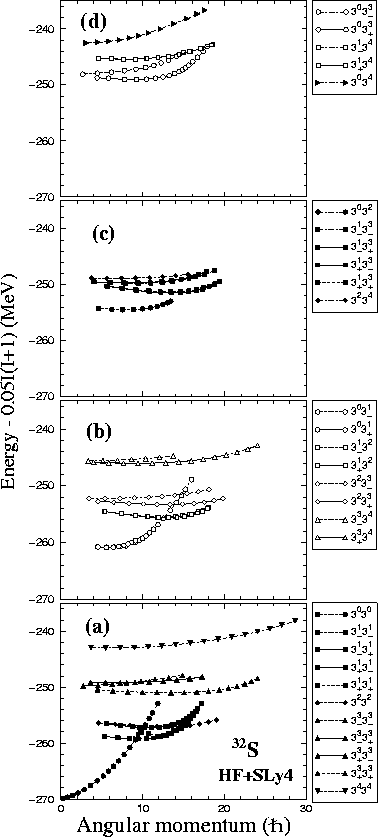Figure 7: Same as in Fig. 6, but for the yrast region of energies.

  

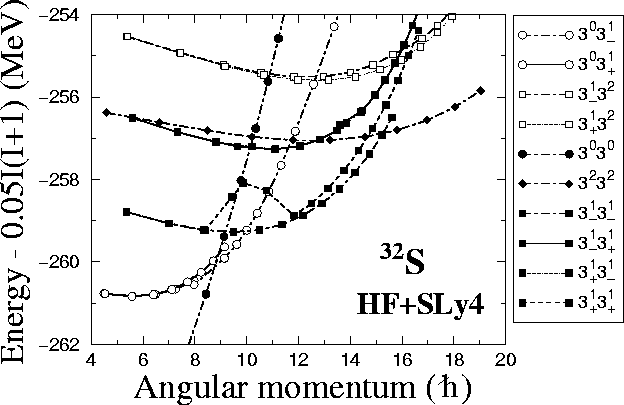Figure: Proton quadrupole moments of the HF bands in ³²S, shown in the form of points on the Q₂₀-Q₂₂ plane. Since the variation of the multipole moments in function of the rotational frequency turns out to be regular the corresponding points form trajectories. Arrows indicate directions of increasing $\hbar\omega$. Note a large difference in scale between the Q₂₀ and Q₂₂axes. The scales were adapted to the large differences between |Q₂₀| and |Q₂₂|. The straight lines corresponding to $\gamma$= $\pm 15^\circ$ and to $\gamma$= $\pm 30^\circ$ have been drawn to facilitate reading the degree of non-axiality of the corresponding solutions.

  

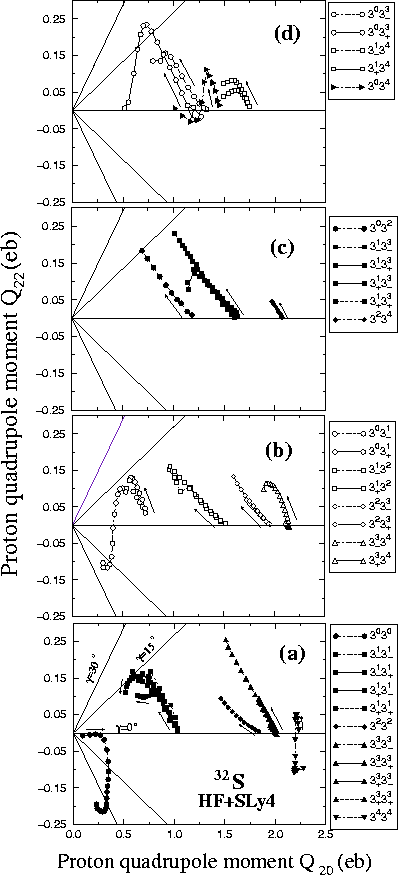Figure: Dynamical moments ${\cal J}^{(2)}$ of the HF bands in ³²S as functions of the rotational frequency. The figure shows results for near-yrast bands selected in Fig. 7.

  

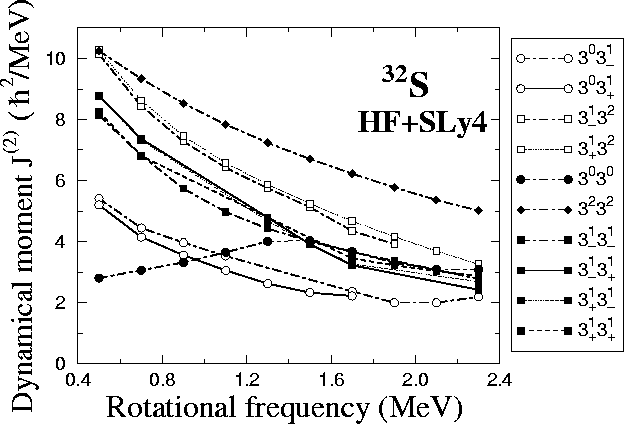Figure: Relative alignments $\delta {I}$ of the HF bands in ³²S as functions of the rotational frequency, calculated with respect to the SD magic band in ³²S. The figure shows results for near-yrast bands selected in Fig. 7.

  

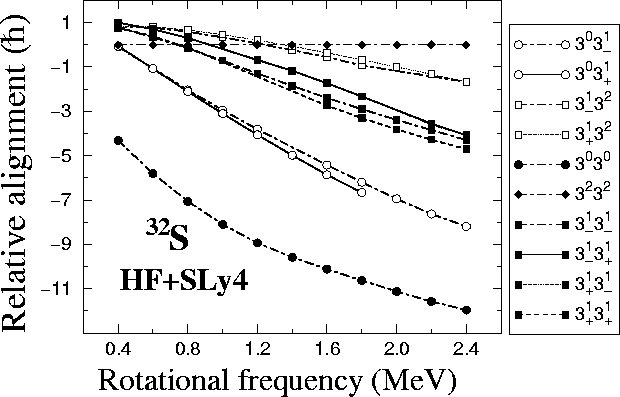Figure: Energies of the HF bands in ³³S and ³¹S as functions of the angular momentum. A rigid-rotor reference energy of 0.05I(I+1)MeV has been subtracted to increase clarity of the plot. Full and open symbols represent the positive- ($\pi$=+1) and negative-parity ($\pi$=-1) bands. Long-short-dashed and solid lines denote signatures r=+i and r=-i, respectively. Configurations 3^1* and 3^3* correspond to the highest negative-parity particles promoted to the next-to-lowest available intruder states. For 3^1*, the first point corresponds to $\hbar\omega$=0.6MeV.

  

Figure 12: Same as in Fig. 11, but for the ³³Cl and ³¹P nuclei.

  

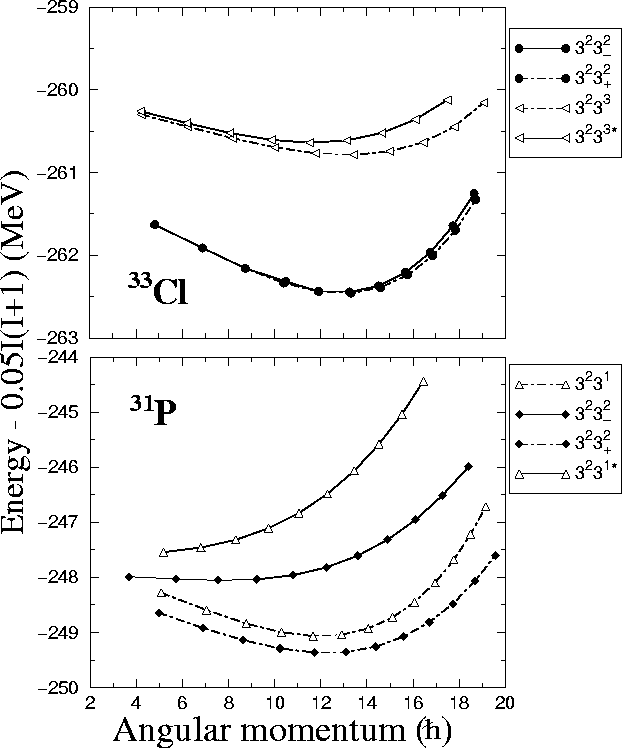Figure: Same as in Fig. 11, but for the dynamical moments ${\cal J}^{(2)}$.

  

Figure 14: Same as in Fig. 13, but for the ³³Cl and ³¹P nuclei.

  

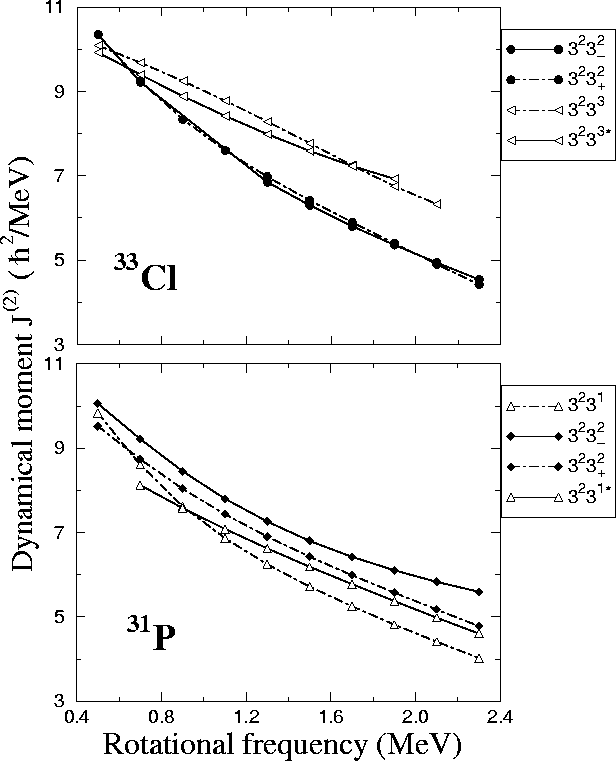Figure: Relative alignments $\delta {I}$ of the HF bands in ³³S, ³¹S, ³³Cl, and ³¹P as functions of the rotational frequency, calculated with respect to the SD magic band in ³²S (see Fig. 11 for conventions used for symbols and lines). The Nilsson labels of particle (p) or hole (h) orbitals, which make the difference between the given band and the magic band in ³²S, are indicated on the right-hand side.

  

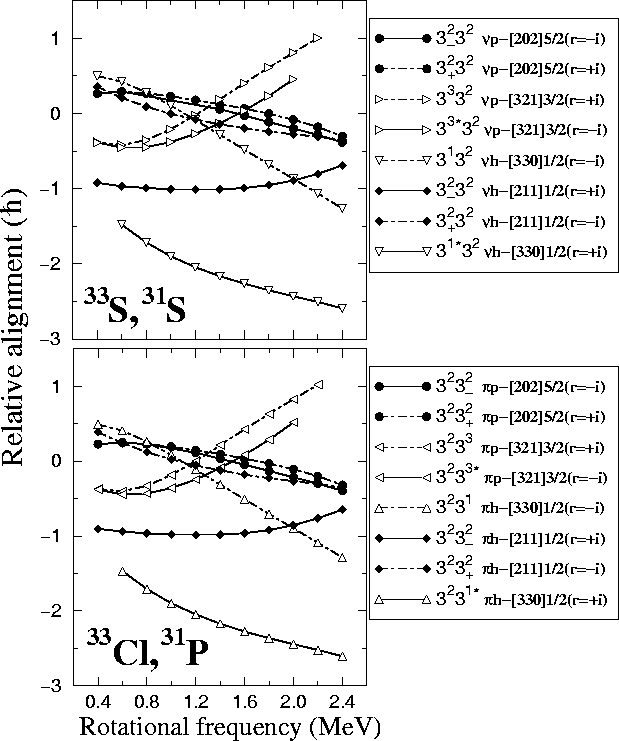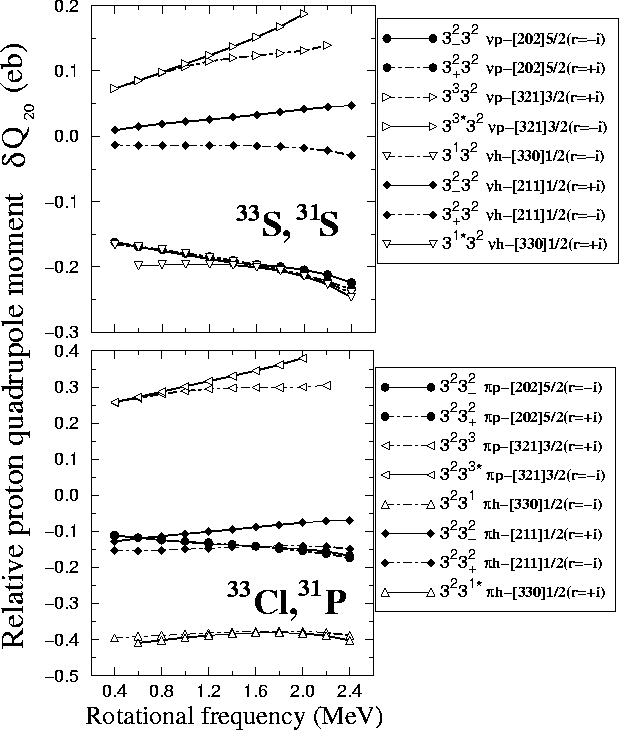
Figure: Same as in Fig. 15, but for the relative proton quadrupole moments $\delta Q_{20}$.