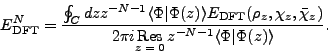Next: Analytic properties
Up: Particle-Number-Projected DFT
Previous: Transition energy density
Projected DFT energy
Based on the above discussion, we postulate the projected DFT energy
in the form:
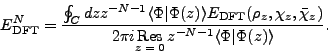 |
(34) |
At variance with the Hamiltonian-based HFB theory, the
projected DFT energy may depend on the integration contour  . Moreover,
the
numerator in Eq. (34) is, in general, not equal to the
residue at
. Moreover,
the
numerator in Eq. (34) is, in general, not equal to the
residue at  =0 as in Eq. (15). Consequently, both the
transition energy density and
the contour
=0 as in Eq. (15). Consequently, both the
transition energy density and
the contour  define the projected
energy in DFT. Since the projected DFT energy (34)
must be real, in view of condition (32),
we restrict further
considerations only to contours which are symmetric with respect to
the real
define the projected
energy in DFT. Since the projected DFT energy (34)
must be real, in view of condition (32),
we restrict further
considerations only to contours which are symmetric with respect to
the real  -axis. Accordingly,
only the upper-half contour
-axis. Accordingly,
only the upper-half contour  above the real axis can be considered
and
above the real axis can be considered
and
![$\oint_C dz\cdots =2{\mbox{\rm\scriptsize {R}}e}[\oint_{C_+} dz\cdots ]$](img112.png) .
.
Jacek Dobaczewski
2007-08-08