Separable Pairing Interaction

The separable finite-range pairing interaction for neutrons ($\tau=n$) and protons ($\tau=p$) that we use in this study is defined as [19]

$$\hat{V}_\tau (\bm{r}_1 s_1, \bm{r}_2 s_2; \bm{r}'_1 s'_1, \bm{r}'_2 s'_2) \hspace*{-3cm} \nonumber$$

$$= - G_\tau \delta (\bm{R}-\bm{R}') P(r) P(r') \frac{1}{2} (1-\hat{P}_{\sigma}) ,$$ (25)

where $\bm{R}=(\bm{r}_1+\bm{r}_2)/2$ denotes the centre of mass coordinate, $\bm{r}=\bm{r}_1-\bm{r}_2$ is the relative coordinate, $r=\vert\bm{r}\vert$, $\hat{P}_{\sigma}$ is the standard spin-exchange operator, and function $P(r)$ is a sum of $m$ Gaussian terms,

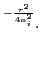$$P(r)=\frac{1}{m} \sum_{i=1}^{m} \frac{1}{(4\pi a_i^2)^{3/2}} ^{-\frac{r^2}{4a_i^2}} .$$ (26)

Coupling constants $G_\tau$ define the pairing strengths for neutrons and protons.

For such a pairing interaction, the pairing energy acquires a fully separable form, which in spherical symmetry reads

$$E_{\text{pair}}^{\text{sep}} = - \frac{1}{2} \sum_{NJ\tau} G_\tau \Bigl( \sum_{\mu \nu} V_{\mu \... ... \langle\psi_{\mu}\vert\vert\kappa'^{+J}_\tau\vert\vert\psi_{\nu}\rangle \Bigr)$$

$$\hspace*{1.5cm}\times\Bigl( \sum_{\mu' \nu'} V_{\mu' \nu'}^{NJ} \langle\psi_{\mu'}\vert\vert\kappa^{J}_\tau\vert\vert\psi_{\nu'} \rangle \Bigr) ,$$ (27)

and depends on the reduced matrix elements of the pairing densities $\kappa_\tau$ and $\kappa'^{+}_\tau$ between the single-particle wave functions $\psi_\mu(\bm{r})$ for $\mu$ denoting the set of spherical harmonic-oscillator quantum numbers $n_\mu l_\mu j_\mu$. The interaction matrix elements $V_{\mu \nu}^{NJ}$ are defined as

$$V_{\mu \nu}^{NJ} = \sqrt{(4\pi)(2J+1)(2j_{\mu}+1)(2j_{\nu}+1)} \begin{Bmatrix}l_{\m... ...u} & J \\ \frac{1}{2} & \frac{1}{2} & 0 \\ j_{\mu} & j_{\nu} & J \end{Bmatrix}$$

$$\times M^{NJn0}_{n_{\mu}l_{\mu}n_{\nu}l_{\nu}} \frac{2^{1/4}}{b^{3/2}} \... ...}(2n+1)!}{2(2^nn!)^2}} \frac{1}{m} \sum_{i=1}^{m} \frac{1}{(4\pi a_i^2)^{3/2}}$$

$$\times \Bigl( \frac{2a_i^2b^2}{1+a_i^2b^2} \Bigr)^{3/2} \Bigl( \frac{1-a_i^2b^2}{1+a_i^2b^2} \Bigr)^{n} ,$$ (28)

where $2n=2n_{\mu}+l_{\mu}+2n_{\nu}+l_{\nu}-2N-J$, $M^{N\lambda n0}_{n_{\mu}l_{\mu}n_{\nu}l_{\nu}}$ are the standard Talmi-Moshinski coefficients [27], and $b=\sqrt{m\omega/\hbar}$ denotes the harmonic-oscillator constant.