Spherical symmetry

In the code HFBRAD the solutions are restricted to have a spherical symmetry and do not mix proton and neutron states. In this situation the wave functions have the good quantum numbers $(n\ell j m q)$ ($n$ is a good quantum number since the spectrum is discretized inside a spherical box) and all the solutions inside an $(n\ell j q)$-block are degenerated. Furthermore, the radial part of the wave functions can be chosen to be real. Thus we use the ansatz

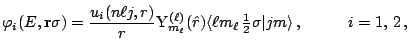$$\varphi_i(E,{\mathbf r}\sigma)=\frac{u_i(n\ell j,r)}{r} {\mathrm ... ...ll m_\ell\,{\textstyle\frac{1}{2}}\sigma\vert jm\rangle\,,\hskip 1cm i=1,\,2\,,$$ (9)

for the wave functions. The local densities can be written using the radial functions (omitting the isospin quantum number)

$$\rho(r) = \displaystyle \frac{1}{4\pi r^2}\sum_{n\ell j}(2j+1)u_2^2(n\ell j,r)\,,\hfill$$ (10)

$$\tilde\rho(r) = \displaystyle -\frac{1}{4\pi r^2}\sum_{n\ell j}(2j+1)u_1(n\ell j,r) u_2(n\ell j,r)\,.\hfill$$ (11)

For the kinetic densities we have

$$\tau(r) = \displaystyle \sum_{n\ell j}\frac{2j+1}{4\pi r^2} \left[ \left(u_... ...l j,r)}{r}\right)^2 +\frac{\ell(\ell+1)}{r^2}u_2^2(n\ell j,r) \right]\,, \hfill$$ (12)

$$\tilde\tau(r) = \displaystyle -\sum_{n\ell j}\frac{2j+1}{4\pi r^2} \left[ \left(u... ...{r}\right) \left(u_2'(n\ell j,r)-\frac{u_2(n\ell j,r)}{r}\right) \right. \hfill$$

$$\hfill\displaystyle +\left.\frac{\ell(\ell+1)}{r^2}u_1(n\ell j,r)u_2(n\ell j,r) \right]\,.$$ (13)

Finally the spin current vector densities have only one non vanishing component given by

$$J(r) = \displaystyle \frac{1}{4\pi r^3}\sum_{n\ell j}(2j+1) \left[j(j+1)-\ell(\ell+1)-\frac{3}{4}\right]u_2^2(n\ell j,r)\,,\hfill$$ (14)

$$\tilde J(r) = \displaystyle -\frac{1}{4\pi r^3}\sum_{n\ell j}(2j+1) \left[j(j+1)-\ell(\ell+1)-\frac{3}{4}\right]u_1(n\ell j,r)u_2(n\ell j,r)\,.$$ (15)