


Next: Spin-orbit and tensor energy
Up: Tensor densities in the
Previous: Tensor densities in the
Momentum-dependent two-body SO[8] and tensor[9,10]
interactions have the form
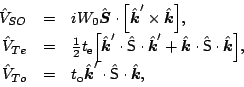 |
(1) |
where the vector and tensor spin operators read
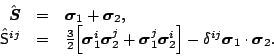 |
(2) |
When averaged with one-body density matrices, these interactions
contribute to the following terms in the EDF (see Refs.[16,4] for
derivations),
![\begin{displaymath}
\begin{array}{rcl}
{\mathcal H}_{SO} &=& {\textstyle{\frac{1...
...}\Big[t_e{J}_n{J}_p
+t_o({J}_0^2-{J}_n{J}_p)\Big] ,
\end{array}\end{displaymath}](img22.png) |
(3) |
where the conservation of time-reversal and spherical symmetries was
assumed. Here,  and
and  are the neutron,
proton, isoscalar, and isovector particle and SO
densities[8,16,4] for
are the neutron,
proton, isoscalar, and isovector particle and SO
densities[8,16,4] for  =
= ,
,  , 0, and
1, respectively.
, 0, and
1, respectively.
Apart from the contribution of the SO
energy density to the central potential, variation of the SO
and tensor terms with respect to the densities yields the one-body
SO potential for neutrons ( =
= ) and protons (
) and protons ( =
= ),
),
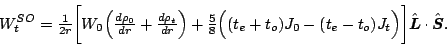 |
(4) |
Hence, it is clear that the only effect of including the tensor
interaction is a modification of the SO splitting of the
single-particle levels, and that, from the point of view of one-body
properties, tensor interactions act very similarly to the two-body
SO interactions. However, the latter ones induce the
SO splitting that is only weakly depending on the shell
filling. This is so because the corresponding form-factor in Eq. (4) is given by the radial derivatives of densities. On the
other hand, the SO splitting induced by the tensor forces
depends strongly on the shell filling, because its form-factor is
given by the SO densities  . Indeed, when only one of
the SO partners is occupied (SUS system), the
SO density is large, and when both partners are occupied
(SS system), the SO density is small, see Sec. 4 for numerical examples.
. Indeed, when only one of
the SO partners is occupied (SUS system), the
SO density is large, and when both partners are occupied
(SS system), the SO density is small, see Sec. 4 for numerical examples.



Next: Spin-orbit and tensor energy
Up: Tensor densities in the
Previous: Tensor densities in the
Jacek Dobaczewski
2006-04-22
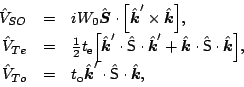
![]() =
=![]() ) and protons (
) and protons (![]() =
=![]() ),
),