A link between the pairing renormalization and regularization procedures

Figure 6: Ratio between the effective pairing strengths for pairing regularization and renormalization, $g_{eff}^{RG}/g_{eff}^{RN}$, for the volume (upper panel) and mixed pairing (lower panel) in $^{120}$Sn for several values of $\epsilon_{\mbox{\rm\scriptsize{cut}}}$.

The renormalized and regularized pairing calculations are based, in fact, on two different effective interactions. Consequently, their results should be comparable only as much as their effective pairing strengths $g_{eff}$ are similar. By expanding Eq. (11) at very high cutoff energies ( $k_F/k_{\mbox{\rm\scriptsize {cut}}}<<1$), one obtains:

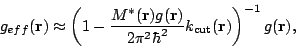$$g_{eff}(\mathbf{r})\approx\left(1-\frac{M^*(\mathbf{r})g(\mat... ...x{\rm\scriptsize {cut}}}(\mathbf{r})\right)^{-1}g(\mathbf{r}),$$ (15)

which has the form of $g_{eff}=\alpha g$. For the volume pairing, the proportionality factor $\alpha$ is $\rho$-dependent only through the weak density dependence of the effective mass $M^*$. On the other hand, for the mixed pairing, it also depends on $\rho$ through the density dependence of $g$. Therefore, while for the volume pairing the renormalization procedure may be considered as a fair approximation to the regularization scheme, this is not the case for the mixed pairing, or - more generally - for any density-dependent pairing. Still, this approximate equality of the effective pairing strengths for the pairing regularization and renormalization is an explanation of the remarkable stability of the total energy in phenomenological pairing renormalization treatment (see Fig. 2), and it also explains why results obtained for the volume pairing are more stable than those in the mixed pairing variant.

This effect can be clearly seen in Fig. 6. The ratio between the effective pairing strengths in the regularization and renormalization methods is much closer to unity for the volume pairing than for the mixed pairing in the region of space, where the pairing energy density is maximal.