The model

The calculations are carried out within the self-consistent constrained Skyrme-Hartree-Fock+BCS (SHF+BCS) framework. The effective Skyrme force SkM$^*$ [9] is used in the particle-hole channel, whereas a seniority pairing force is taken in the particle-particle channel to describe nuclear superfluidity. The seniority pairing force is treated within the BCS procedure, with the strength parameters defined as in Ref. [10]:

$$\begin{array}{l} G^{n}=\left[19.3-0.084 \left( N-Z \right)\r... ...=\left[13.3+0.217 \left( N-Z \right)\right]/A\,,\\ \end{array}$$ (1)

and additionally scaled by

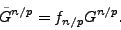$$\tilde{G}^{n/p}= f_{n/p}G^{n/p}.$$ (2)

The scaling factors of Eq. (2), $f_{n}=1.28$ and $f_{p}=1.11$, were adjusted to reproduce the experimental [11] neutron ( $\Delta_{n}=0.696$ MeV) and proton ( $\Delta_{p}=0.803$ MeV) pairing gaps in $^{252}$Fm. The pairing-active space consisted of the lowest $Z$ ($N$) proton (neutron) single-particle states.

The self-consistent HF+BCS equations were solved using the code HFODD (v.2.25b) [12,13,14] that employs the Cartesian 3D deformed harmonic-oscillator finite basis. In the calculations, we took the lowest 1140 single-particle states for the basis. This corresponds to 17 spherical oscillator shells.

Figure: The total binding energy $E^{\mbox{\scriptsize{tot}}}$ (left axis) and mass hexadecapole moment $Q_{40}$ (right axis) along the calculated symmetric compact (sCF), symmetric elongated (sEF), and asymmetric elongated (aEF) fission paths in $^{256}$Fm. Differences in $E^{\mbox{\scriptsize{tot}}}$ shown in the vicinity of the first (inner) barrier illustrate the effect of triaxiality.