The no-core configuration-interaction model

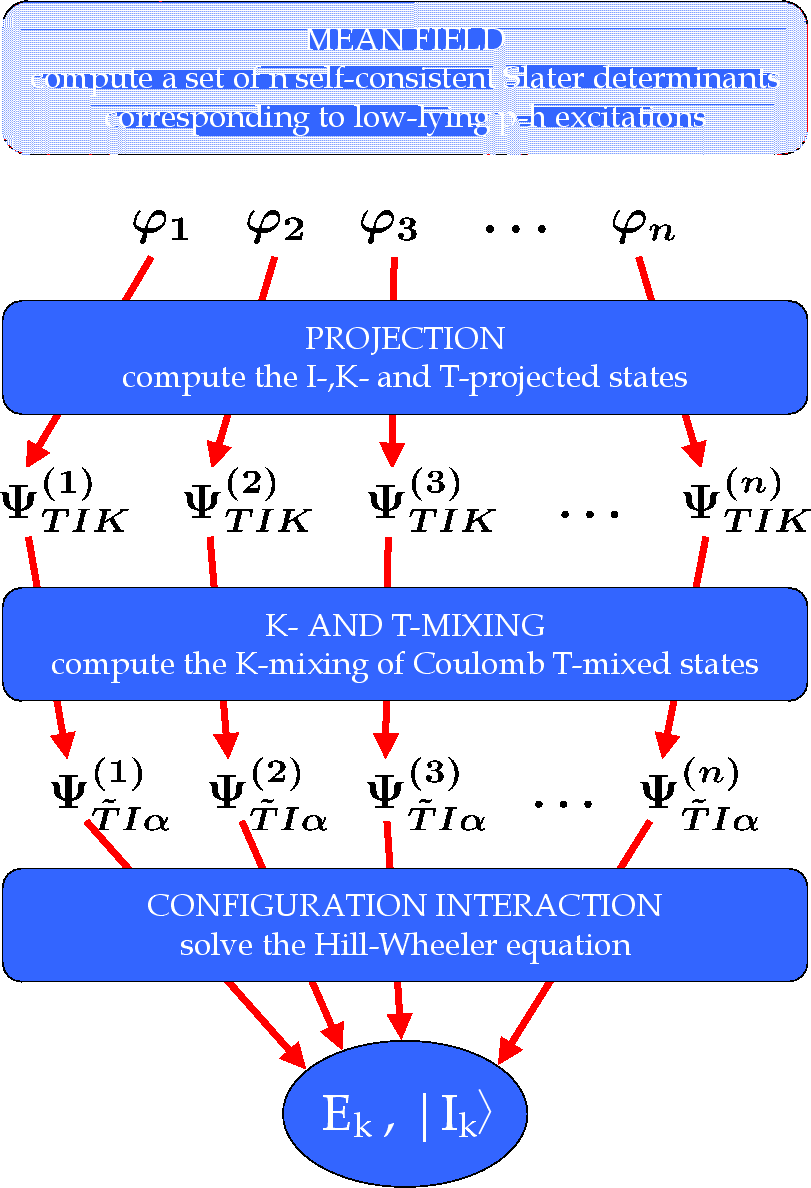
Figure 1: (Color online) Computational scheme of the NCCI model. See text for details.

The static model developed in our previous works [15,21] involved the isospin and angular momentum projections (after variation) of a single Slater determinant, followed by a rediagonalization of the Coulomb force, so as to account properly for the isospin mixing. Here we extend the model towards a variant, in which we allow for a mixing of states projected from different low-lying (multi)particle-(multi)hole Slater determinants $\varphi_i$ with the mixing matrix elements derived from the same Hamiltonian that is used to calculate them.

The computational scheme of our NCCI model is sketched in Fig. 1. It proceeds in four major steps:

• First, a set of relevant low-lying (multi)particle-(multi)hole HF states $\{ \varphi_i \}$ is calculated along with their HF energies $e^{{\rm (HF)}}_i$. States $\{ \varphi_i \}$ form a subspace of reference states for subsequent projections.

• Second, the projection techniques are applied to the set of reference states $\{ \varphi_i \}$, so as to determine the family of states $\{\Psi_{TIK}^{(i)}\}$ having good isospin $T$, angular momentum $I$, and angular-momentum projection on the intrinsic axis $K$.

• Third, states $\{\Psi_{TIK}^{(i)}\}$ are mixed, so as to properly take into account the $K$ mixing and Coulomb isospin mixing - this gives the set of good angular-momentum states $\{\Psi_{\tilde{T}I\alpha}^{(i)}\}$ of the static model [14,15]. Here we label them with the dominating values of the isospin, $\tilde{T}$, and auxiliary quantum numbers $\alpha$. Note that in this step, the mixing is performed for each configuration $i$ separately (the static model).

• Finally, the results of the dynamic model correspond to mixing non-orthogonal states $\{\Psi_{\tilde{T}I\alpha}^{(i)}\}$ for all configurations $i$, and for all values of $\tilde{T}$ and $\alpha$. This is performed by solving the Hill-Wheeler equation ${\cal{H}}\vert I_k\rangle=E_k{\cal{N}}\vert I_k\rangle$ [22] in the collective space spanned by the natural states corresponding to sufficiently large eigenvalues of the norm matrix ${\cal{N}}$. This is the same technique that is used in the code to handle the $K$-mixing alone. The method is described in details in Ref. [23].

We note here that all wave functions considered above correspond to good neutron ($N$), and proton ($Z$) numbers, and thus to a good third component of the isospin, $T_z=\tfrac{1}{2}(N-Z)$. We also note that the configuration interaction, which is taken into account in the last step, could have also equivalently been performed by directly mixing the projected states $\{\Psi_{TIK}^{(i)}\}$. The procedure outlined above simply aims to obtain separately the results of the static and dynamic model. The NCCI calculations discussed below were performed using a new version of the HFODD solver [24], which was equipped with the NCCI module. This new implementation was based on the previous versions of the code [23,25,26].

Numerical stability of the method depends on necessary truncations of the model space. In this work, numerically unstable solutions are removed by truncating the natural states corresponding to small eigenvalues of the norm matrix $N$. It means that only the natural states corresponding to the eigenvalues of the norm matrix that are larger than certain externally provided cut-off parameter $\zeta$ are used to built the so-called collective space. Although such a truncation procedure gives reliable values of the energy, a full stability of the method still requires further studies. Other methods, e.g., based on truncating high-energy states $\{\Psi_{\tilde{T}I\alpha}^{(i)}\}$, or combined methods involving both truncations simultaneously, need to be studied as well.